3.5 Deterministic Chaos

“The study of things that look random -but are not”

—E. Lorenz

[This Google sheet]((https://docs.google.com/spreadsheets/d/1xaZJLYfZzkxg_PXCAa7QT7p4R_JTnUIxzs_Qs1-RAOQ/edit?usp=sharing) displays the extreme sensitivity to changes in initial conditions displayed by the Logistic Map for specific parameter settings. This specific phenomenon is more commonly referred to as The Butterfly Effect. It is a characteristic of a very interesting and rather mysterious behaviour displayed by deterministic dynamical equations known as deterministic chaos.

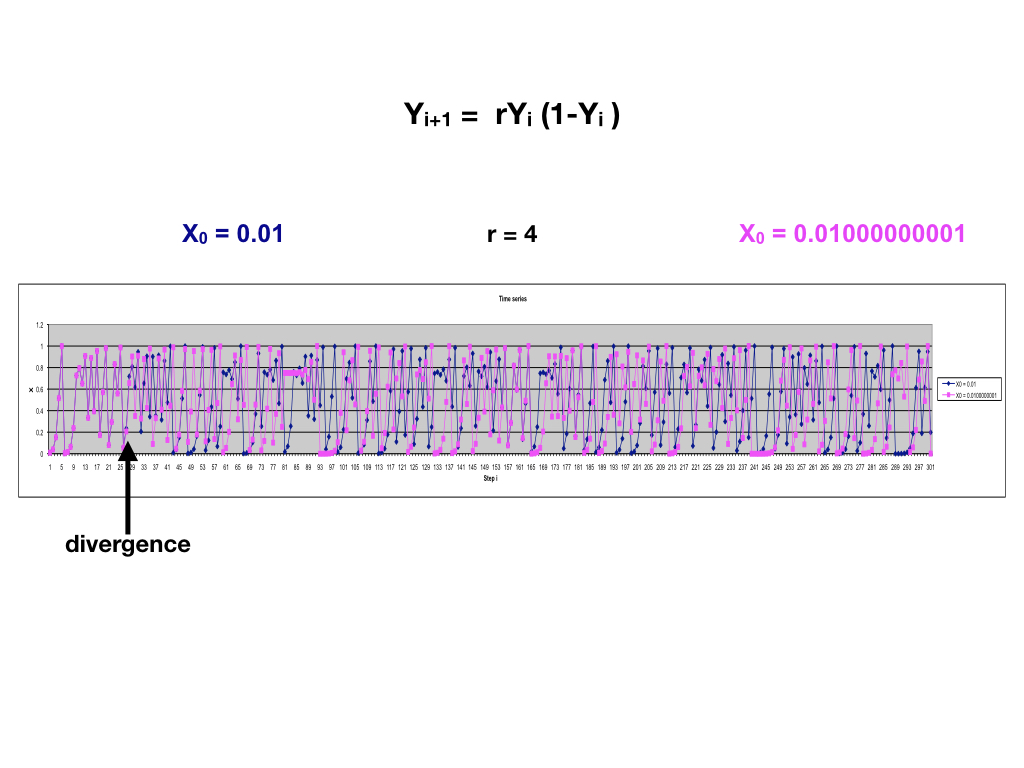
Figure 3.2: Butterfly Effect

The Logistic Map is the simplest nontrivial model that can display deterministic chaos. For continuous time models, one needs at least 3 coupled differential equations to generate chaotic behavior. There is no clear definition of deterministic chaos, but there are at least 4 ingredients: The dynamics are a-periodic, bounded and sensitively depend on initial conditions, moreover, the system generating these dynamics is deterministic.

The video below by YouTuber Veritasium does an amazing job at explaining what deterministic chaos is based on the famous Lorenz system.

The science behind the butterfly effect